10. Image Credits

1. Motivation and Introduction

Fruits
A bowl of fruit
Thomas Severiens
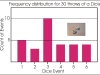
Frequency distribution for 30 throws of a dice
Two dice / Frequency distribution for 30 throws of a dice
Keith R. McCloy
Frequency distribution for a sample of 754 school students
Frequency distribution for a sample of 754 school students
Keith R. McCloy
Landsat image of Middelfart - Fredericia, Denmark
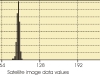
A false colour composite image of the Middelfart - Fredericia area of Denmark, acquired by the Landsat TM satellite
Date of aquisition: 03.06.2004
Image source: USGS; image processing: Keith R. McCloy

2. Mathematical Methods

Frequency distribution for 30 throws of a dice
One dice / Frequency distribution for 30 throws of a dice
Keith R. McCloy and Thomas Severiens
Frequency distribution for a sample of 754 school students
Frequency distribution for a sample of 754 school students
Keith R. McCloy
A frequency distribution for one training area on one cover type
A frequency distribution for one training area on one cover type
Keith R. McCloy
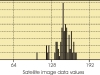
A frequency distribution for one training area on another cover type
A frequency distribution for one training area on another cover type
Keith R. McCloy
Frequency distributions for the whole of the Skagen image and for the three training areas
Frequency distributions for the whole of the Skagen image and for the three training areas
Keith R. McCloy
Landsat TM5 image of the Skagen area of Denmark
Landsat TM5 image of the Skagen area of Denmark
Date of aquisition: 03.06.2004
Image source: USGS; image processing: Keith R. McCloy
Sample distribution
Sample distribution
Keith R. McCloy
Landsat TM5 image of the Skagen area of Denmark
Landsat TM5 image of the Skagen area of Denmark
Date of aquisition: 03.06.2004
Image source: USGS; image processing: Keith R. McCloy
Scattergrams
Scattergrams for bands 1 and 2 on the left and bands 1 and 4 on the right for the image above
Keith R. McCloy
Two dice
One dice
Thomas Severiens
Possible outcome of two throws of a dice
Possible outcome of two throws of a dice
Keith R. McCloy
Horse Racing
Horse Racing: Galopp Riem 05.06.2005, Munich, Germany
Softeis
Wikimedia Commons
Last access: 31.01.2009
Histogram of throws of dice
Histogram of throws of dice
Keith R. McCloy
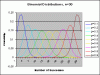
Binomial Distribution
Binomial Distribution for n = 30 showing how the shape changes as the probability of an event changes
Keith R. McCloy
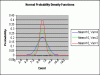
Underwater Litter
Normal Probability Distribution Functions
Keith R. McCloy

3. What is Classification?

Typical scattergrams for 4 band satellite image data
Typical scattergrams for 4 band satellite image data
Keith R. McCloy
The process of classification I
The process of classification I
Keith R. McCloy
The process of classification II
The process of classification II
Keith R. McCloy
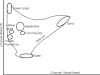
The physical meaning of land cover classes
The physical meaning of the land cover classes depicted in one of the scattergrams depicted on this page
Keith R. McCloy

4. The Minimum Distance Classifier

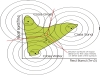
Three classes, their Euclidean Distances and their Minimum Distance Decision Surfaces
Three classes, their Euclidean Distances and their Minimum Distance Decision Surfaces
Keith R. McCloy
Skagen image, training areas and Minimum Distance Classification
Skagen image, training areas and Minimum Distance Classification
Image source: USGS; image processing: Keith R. McCloy

5. The Maximum Likelihood Classifier

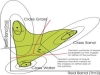
Scattergram
Scattergram depicting three class means, their distribution in units of standard deviation, and class decision surfaces
Keith R. McCloy
Skagen area, minimum distance and maximum likelihood classification
Skagen area, minimum distance and maximum likelihood classification
Image source: USGS; image processing: Keith R. McCloy

6. Errors and Costs in Classification

Two possible decision surfaces
Two classes in one dimension, their normal distributions and two possible decision surfaces that could be sued to discriminate between objects in the two classes
Keith R. McCloy